Eksempler på binær til Ascii-konvertering
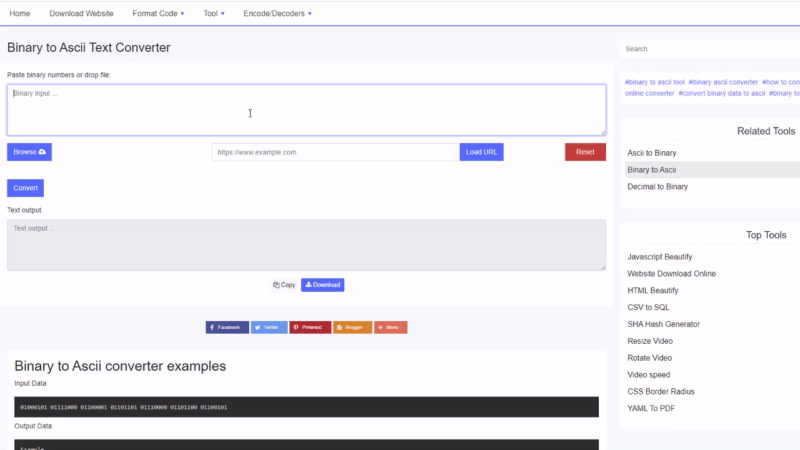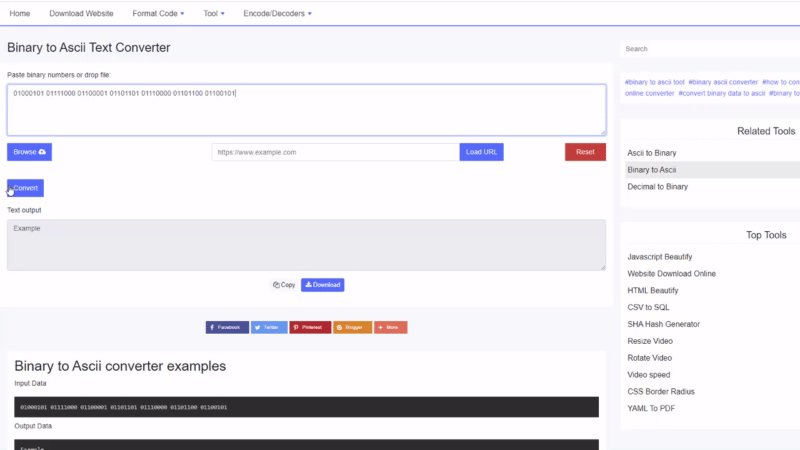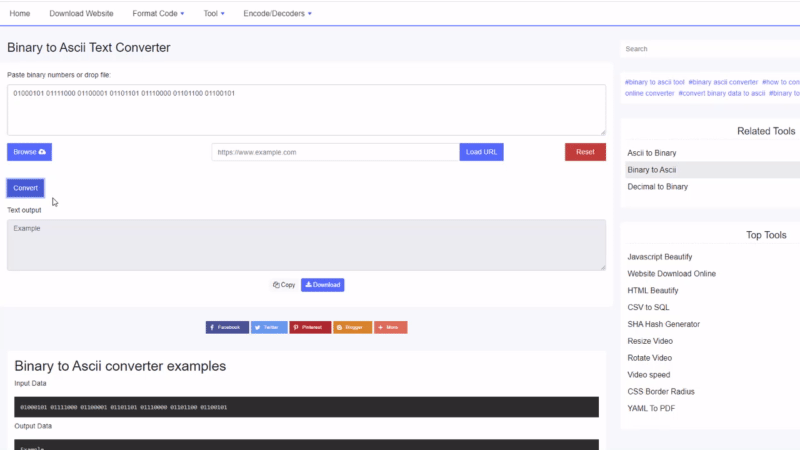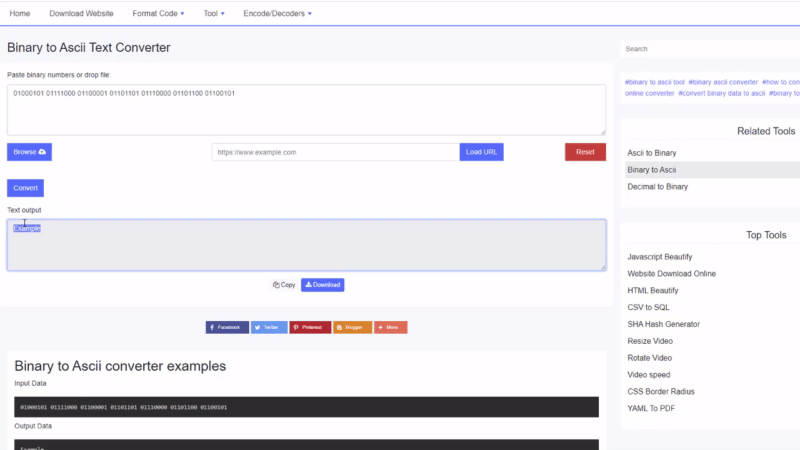
Inndata
01000101 01111000 01100001 01101101 01110000 01101100 01100101Utdata
ExampleHvordan konvertere binær til tekst
Konverter binær ASCII-kode til tekst:
- Få binær byte
- Konverter binær byte til desimal
- Få tegn til ASCII-kode fra ASCII-tabellen
- Fortsett med neste byte
Hvordan konvertere 01000001 binær til tekst?
Bruk ASCII-tabell:
010100002 = 26+24 = 64+16 = 80 => "P"
011011002 = 26+25+23+22 = 64+32+8+4 = 108 => "l"
011000012 =+ 26 20 = 64+32+1 = 97 => "a"
01000001 = 2^6+2^2 = 64+1 = 65 = 'A'
00110000 = 2^5+2^4 = 2^5+2^4 = 32+16 = 48 = '0'
Binær til ASCII tekstkonverteringstabell
| Heksadesimal | Binær | ASCII-karakter |
|---|---|---|
| 00 | 00000000 | NUL |
| 01 | 00000001 | SOH |
| 02 | 00000010 | STX |
| 03 | 00000011 | ETX |
| 04 | 00000100 | EOT |
| 05 | 00000101 | ENQ |
| 06 | 00000110 | ACK |
| 07 | 00000111 | BEL |
| 08 | 00001000 | BS |
| 09 | 00001001 | HT |
| 0A | 00001010 | LF |
| 0B | 00001011 | VT |
| 0C | 00001100 | FF |
| 0D | 00001101 | CR |
| 0E | 00001110 | SÅ |
| 0F | 00001111 | SI |
| 10 | 00010000 | DLE |
| 11 | 00010001 | DC1 |
| 12 | 00010010 | DC2 |
| 1. 3 | 00010011 | DC3 |
| 14 | 00010100 | DC4 |
| 15 | 00010101 | NAK |
| 16 | 00010110 | SYN |
| 17 | 00010111 | ETB |
| 18 | 00011000 | KAN |
| 19 | 00011001 | EM |
| 1A | 00011010 | UNDER |
| 1B | 00011011 | ESC |
| 1C | 00011100 | FS |
| 1D | 00011101 | GS |
| 1E | 00011110 | RS |
| 1F | 00011111 | OSS |
| 20 | 00100000 | Rom |
| 21 | 00100001 | ! |
| 22 | 00100010 | " |
| 23 | 00100011 | # |
| 24 | 00100100 | $ |
| 25 | 00100101 | % |
| 26 | 00100110 | & |
| 27 | 00100111 | ' |
| 28 | 00101000 | ( |
| 29 | 00101001 | ) |
| 2A | 00101010 | * |
| 2B | 00101011 | + |
| 2C | 00101100 | , |
| 2D | 00101101 | - |
| 2E | 00101110 | . |
| 2F | 00101111 | / |
| 30 | 00110000 | 0 |
| 31 | 00110001 | 1 |
| 32 | 00110010 | 2 |
| 33 | 00110011 | 3 |
| 34 | 00110100 | 4 |
| 35 | 00110101 | 5 |
| 36 | 00110110 | 6 |
| 37 | 00110111 | 7 |
| 38 | 00111000 | 8 |
| 39 | 00111001 | 9 |
| 3A | 00111010 | : |
| 3B | 00111011 | ; |
| 3C | 00111100 | < |
| 3D | 00111101 | = |
| 3E | 00111110 | > |
| 3F | 00111111 | ? |
| 40 | 01000000 | @ |
| 41 | 01000001 | A |
| 42 | 01000010 | B |
| 43 | 01000011 | C |
| 44 | 01000100 | D |
| 45 | 01000101 | E |
| 46 | 01000110 | F |
| 47 | 01000111 | G |
| 48 | 01001000 | H |
| 49 | 01001001 | I |
| 4A | 01001010 | J |
| 4B | 01001011 | K |
| 4C | 01001100 | L |
| 4D | 01001101 | M |
| 4E | 01001110 | N |
| 4F | 01001111 | O |
| 50 | 01010000 | P |
| 51 | 01010001 | Q |
| 52 | 01010010 | R |
| 53 | 01010011 | S |
| 54 | 01010100 | T |
| 55 | 01010101 | U |
| 56 | 01010110 | V |
| 57 | 01010111 | W |
| 58 | 01011000 | X |
| 59 | 01011001 | Y |
| 5A | 01011010 | Z |
| 5B | 01011011 | [ |
| 5C | 01011100 | \ |
| 5D | 01011101 | ] |
| 5E | 01011110 | ^ |
| 5F | 01011111 | _ |
| 60 | 01100000 | ` |
| 61 | 01100001 | a |
| 62 | 01100010 | b |
| 63 | 01100011 | c |
| 64 | 01100100 | d |
| 65 | 01100101 | e |
| 66 | 01100110 | f |
| 67 | 01100111 | g |
| 68 | 01101000 | h |
| 69 | 01101001 | i |
| 6A | 01101010 | j |
| 6B | 01101011 | k |
| 6C | 01101100 | l |
| 6D | 01101101 | m |
| 6E | 01101110 | n |
| 6F | 01101111 | o |
| 70 | 01110000 | p |
| 71 | 01110001 | q |
| 72 | 01110010 | r |
| 73 | 01110011 | s |
| 74 | 01110100 | t |
| 75 | 01110101 | u |
| 76 | 01110110 | v |
| 77 | 01110111 | w |
| 78 | 01111000 | x |
| 79 | 01111001 | y |
| 7A | 01111010 | z |
| 7B | 01111011 | { |
| 7C | 01111100 | | |
| 7D | 01111101 | } |
| 7E | 01111110 | ~ |
| 7F | 01111111 | DEL |
Binary System
The binary numeral system uses the number 2 as its base (radix). As a base-2 numeral system, it consists of only two numbers: 0 and 1.
While it has been applied in ancient Egypt, China and India for different purposes, the binary system has become the language of electronics and computers in the modern world. This is the most efficient system to detect an electric signal’s off (0) and on (1) state. It is also the basis for binary code that is used to compose data in computer-based machines. Even the digital text that you are reading right now consists of binary numbers.
ASCII Text
ASCII (American Standard Code for Information Interchange) er en av de vanligste standardene for tegnkoding. Opprinnelig utviklet fra telegrafiske koder, er ASCII nå mye brukt i elektronisk kommunikasjon for å formidle tekst.
Den originale ASCII er basert på 128 tegn. Dette er de 26 bokstavene i det engelske alfabetet (både i små og store bokstaver); tall fra 0 til 9; og ulike skilletegn. I ASCII-koden er hvert av disse tegnene tildelt et desimaltall fra 0 til 127. For eksempel er ASCII-representasjonen av store bokstaver A 65 og små bokstaver a er 97.