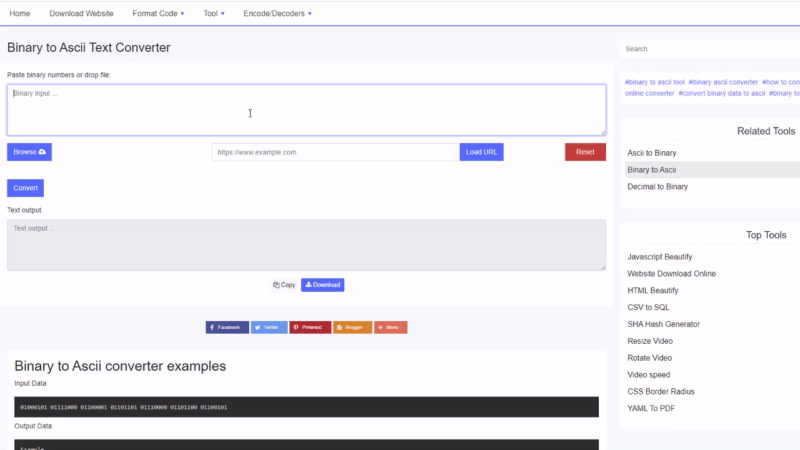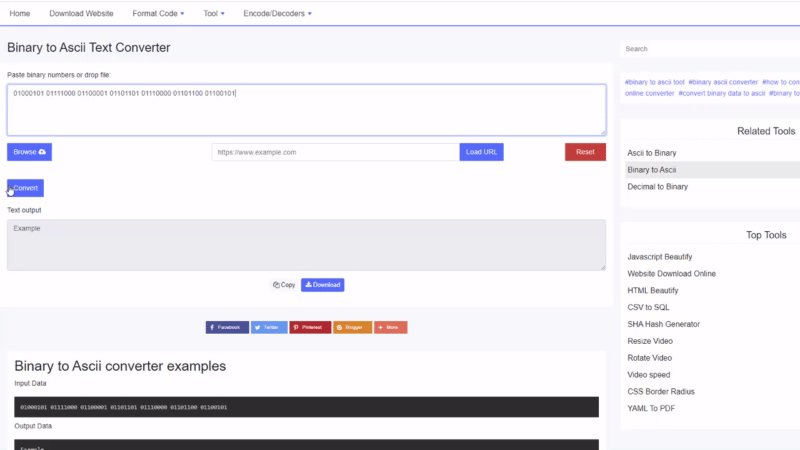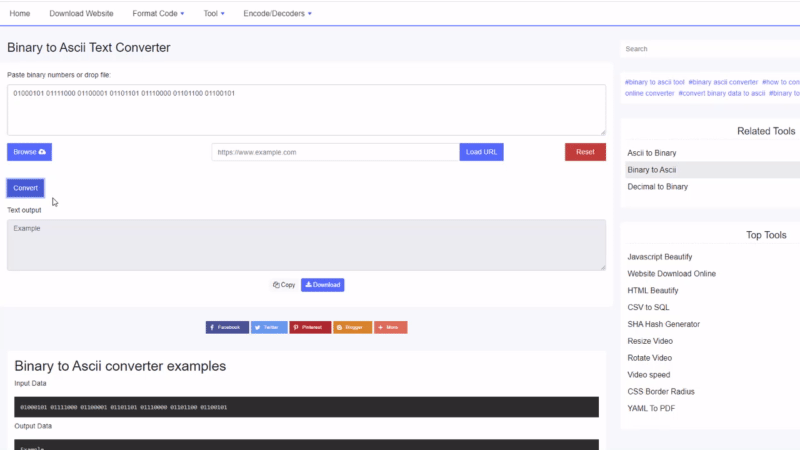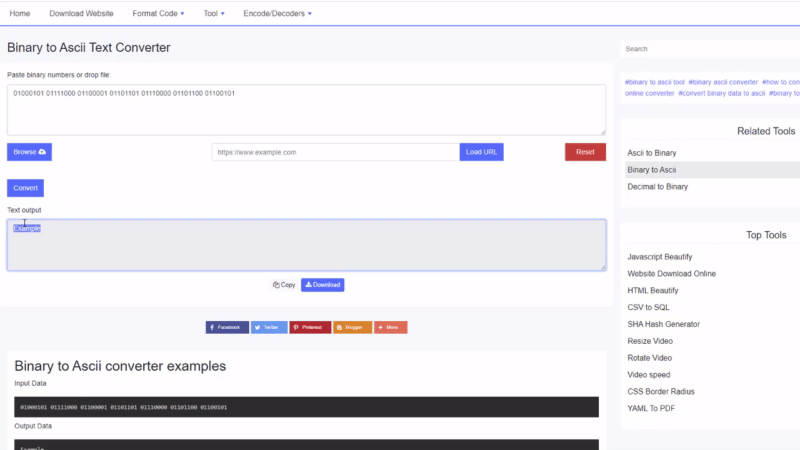
Binär till Ascii Text Converter

Paste binary numbers or drop file:

Browse

Text output

Exempel på binära till Ascii-omvandlare

Indata

01000101 01111000 01100001 01101101 01110000 01101100 01100101

Utdata

Example

Hur man konverterar binär till text

Konvertera binär ASCII-kod till text:

Hämta binär byte
Konvertera binär byte till decimal
Hämta tecken för ASCII-kod från ASCII-tabellen
Fortsätt med nästa byte

Hur konverterar man 01000001 binär till text?

Använd ASCII-tabell:
010100002 = 26+24 = 64+16 = 80 => "P"
011011002 = 26+25+23+22 = 64+32+8+4 = 108 => "l" 0110000012 2 =
0 = 64+32+1 = 97 => "a"
01000001 = 2^6+2^2 = 64+1 = 65 = 'A'
00110000 = 2^5+2^4 = 2^5+2^4 = 32+16 = 48 = '0'

Binär till ASCII-textkonverteringstabell

Hexadecimal	Binär	ASCII-tecken
00	00000000	NUL
01	00000001	SOH
02	00000010	STX
03	00000011	ETX
04	00000100	EOT
05	00000101	ENQ
06	00000110	ACK
07	00000111	BEL
08	00001000	BS
09	00001001	HT
0A	00001010	LF
0B	00001011	VT
0C	00001100	FF
0D	00001101	CR
0E	00001110	SÅ
0F	00001111	SI
10	00010000	DLE
11	00010001	DC1
12	00010010	DC2
13	00010011	DC3
14	00010100	DC4
15	00010101	NAK
16	00010110	SYN
17	00010111	ETB
18	00011000	BURK
19	00011001	EM
1A	00011010	SUB
IB	00011011	ESC
1C	00011100	FS
1D	00011101	GS
1E	00011110	RS
1F	00011111	USA
20	00100000	Plats
21	00100001	!
22	00100010	"
23	00100011	#
24	00100100	$
25	00100101	%
26	00100110	&
27	00100111	'
28	00101000	(
29	00101001	)
2A	00101010	*
2B	00101011	+
2C	00101100	,
2D	00101101	-
2E	00101110	.
2F	00101111	/
30	00110000	0
31	00110001	1
32	00110010	2
33	00110011	3
34	00110100	4
35	00110101	5
36	00110110	6
37	00110111	7
38	00111000	8
39	00111001	9
3A	00111010	:
3B	00111011	;
3C	00111100	<
3D	00111101	=
3E	00111110	>
3F	00111111	?
40	01000000	@
41	01000001	A
42	01000010	B
43	01000011	C
44	01000100	D
45	01000101	E
46	01000110	F
47	01000111	G
48	01001000	H
49	01001001	I
4A	01001010	J
4B	01001011	K
4C	01001100	L
4D	01001101	M
4E	01001110	N
4F	01001111	O
50	01010000	P
51	01010001	Q
52	01010010	R
53	01010011	S
54	01010100	T
55	01010101	U
56	01010110	V
57	01010111	W
58	01011000	X
59	01011001	Y
5A	01011010	Z
5B	01011011	[
5C	01011100	\
5D	01011101	]
5E	01011110	^
5F	01011111	_
60	01100000	`
61	01100001	a
62	01100010	b
63	01100011	c
64	01100100	d
65	01100101	e
66	01100110	f
67	01100111	g
68	01101000	h
69	01101001	i
6A	01101010	j
6B	01101011	k
6C	01101100	l
6D	01101101	m
6E	01101110	n
6F	01101111	o
70	01110000	p
71	01110001	q
72	01110010	r
73	01110011	s
74	01110100	t
75	01110101	u
76	01110110	v
77	01110111	w
78	01111000	x
79	01111001	y
7A	01111010	z
7B	01111011	{
7C	01111100	\|
7D	01111101	}
7E	01111110	~
7F	01111111	DEL

Binary System

The binary numeral system uses the number 2 as its base (radix). As a base-2 numeral system, it consists of only two numbers: 0 and 1.

While it has been applied in ancient Egypt, China and India for different purposes, the binary system has become the language of electronics and computers in the modern world. This is the most efficient system to detect an electric signal’s off (0) and on (1) state. It is also the basis for binary code that is used to compose data in computer-based machines. Even the digital text that you are reading right now consists of binary numbers.

ASCII Text

ASCII (American Standard Code for Information Interchange) är en av de vanligaste teckenkodningsstandarderna. ASCII, som ursprungligen utvecklades från telegrafiska koder, används nu flitigt i elektronisk kommunikation för att förmedla text.

Den ursprungliga ASCII är baserad på 128 tecken. Dessa är de 26 bokstäverna i det engelska alfabetet (både i gemener och versaler); siffror från 0 till 9; och olika skiljetecken. I ASCII-koden tilldelas vart och ett av dessa tecken ett decimaltal från 0 till 127. Till exempel är ASCII-representationen av versaler A 65 och gemena a är 97.